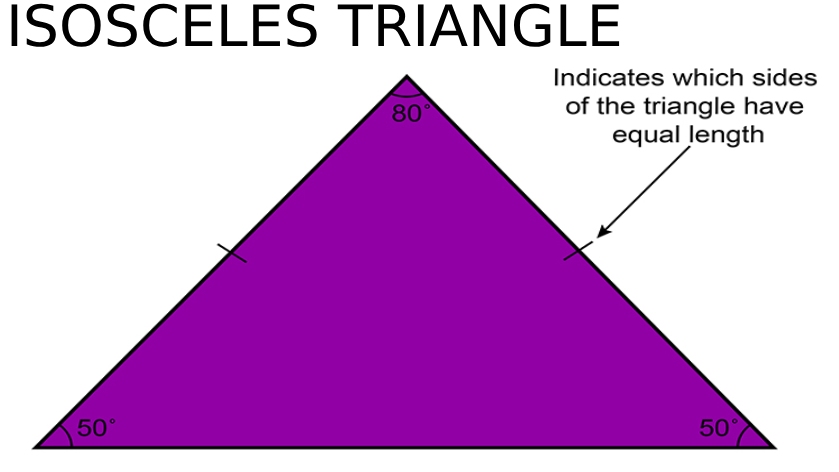
A triangle with two sides of equivalent length is an isosceles triangle.
If sides AB and AC are identical in a triangle ABC, then △ ABC is an isosceles triangle everywhere ∠ B = ∠ C. The theorem that defines the isosceles triangle is “if the dual sides of a triangle are corresponding, then the angle opposite to them are also congruent.”
Table of Contents
Define Isosceles Triangle
An isosceles triangle is a kind of triangle that has any two sides equivalent in length. The two angles of an isosceles triangle are similar, conflicting to equal sides. In geometry, the triangle is a three-sided polygonal shape that is divided into three categories based on its sides,
Examples of Isosceles Triangle
Parts of an Isosceles Triangles
Hind leg
The two equal edges of an isosceles triangle are identified as ‘legs. In this triangle, ABC (given above), AB, and AC are the 2 sides of the isosceles triangle.
Sordid
The ‘base’ of an isosceles triangle is the third and incapable side. In the triangle, ABC, BC is the bottom line of the isosceles triangle.
Vertex angle
The ‘vertex angle’ is formed by two equal sides of an isosceles triangle. ∠BAC is the vertex angle of the isosceles triangle.
Bottom line sides
The ‘bottom line angles’ are the angles that include the base of an isosceles triangle. ∠ABC and ∠ACB are the 2 base angles of the isosceles triangle.
Properties of Isosceles Triangle
The unequal side is called the triangle’s base because the two sides are equal in this triangle.
The angles opposite to the 2 equal sides of the triangle are always equivalent.
The elevation of an isosceles triangle is measured from the base to the topmost of the triangle.
A right isosceles triangle has a 3rd angle of 90 degrees
In an isosceles triangle, if two sides are equivalent, the angles opposite to the two sides correspond to each other and are always equal.
The two angles ∠, B and ∠C, contradictory to the equal sides AB and AC, are similar in the isosceles triangle above.
The isosceles triangle has three acute angles, which means the angles are less than 90 degrees.
The summation of three angles of an isosceles triangle is constantly 180 degrees.
Types of Isosceles Triangles
Usually, the isosceles triangle is classified into different types, namely,
- Isosceles acute triangle
- Isosceles right triangle
- Isosceles obtuse triangle
Now, let us discuss these three types of isosceles triangles in detail.
Isosceles acute triangle
As we know, a triangle’s dimensions are legs, base, and height. All the isosceles triangles have an axis of symmetry along the perpendicular bisector of their base. The isosceles triangle is categorized as acute, right, and imperceptive, depending on the angle between the two sides. The isosceles triangle can be critical if the two angles opposite the legs are identical and are less than 90 degrees (sharp angles).
Isosceles right triangles
The right isosceles triangle has two equal sides, where one of the two equal sides acts perpendicularly, and the other acts as a bottom line of the triangle. The third side, which is unfit, is termed the hypotenuse. Therefore, we can apply the famed Pythagoras theorem, where the square of the hypotenuse is equivalent to the sum of the square of the base and vertical.
Assume the sides of the right isosceles triangle are a, a, and h, wherever a is the two equivalent sides, and h is the hypotenuse. Then
h = (a2 + a2) = √2a2 = a√2 or h = √2 a
Isosceles obtuse triangles
An isosceles obtuse triangles are the triangles in which one of the 3 angles is obtuse (lies between 90° and 180°), and the additional two acute angles are equivalent in size. One illustration of isosceles obtuse triangle angles is 30°, 30°, and 120°.
Area and Perimeter of Isosceles Triangle
The following formula gives the area of an isosceles triangle:
Area (A) = ½ × base (b) × height (h)
The formula gives the perimeter of the isosceles triangle:
Perimeter(P) = 2a + base(b)
Here, ‘a’ denotes the length of the equivalent sides of the isosceles triangle, and ‘b’ mentions the length of the third unequal side.
Perimeter of Isosceles Triangles
As we know, the perimeter of any shape is the shape’s boundary. Likewise, the perimeter of an isosceles triangle is well-defined as the three sides of an isosceles triangle. The perimeter of an isosceles triangle can originate if we know its base and sides. The formula to compute the perimeter of the isosceles triangle is given below:
The perimeters of an isosceles Triangle, P = 2a + b unit
Where ‘a’ is the length of the two equal sides of an isosceles triangle, and b is the triangle’s base.
Isosceles Triangle Theorem
As per the theorem, if two sides correspond in an isosceles triangle, then the angles opposite to the two sides are also harmonious.
Instead, if two angles are congruent in an isosceles triangle, the sides opposite to them are also melodious.
In the above triangle ABC,
AB = AC
∠ABC = ∠ADC
Angles of Isosceles Triangles
The two of the three angles of the isosceles triangles are equal in measure, opposites to the equal sides. Hence, one of the angles is unsatisfactory. Assume that if the measurements of an unequal angle are given to us, then we can effortlessly find the other two angles by the angle of the sum property.
Example: Given an isosceles triangle.
Let the measure of the unequal angle be 70°, and the other two equivalent angles measure x; then, as per the angle sum rule,
70° + x + x = 180°
70° + 2x = 180°
2x = 180 – 70 = 110°
x = 110/2 = 55°
Hence, the measure of the other two angles of an isosceles triangle is 55°.
Discuss two essential properties of an isosceles triangle.
The angles adjacent to the two equal sides of a triangle are also similar.
The two equivalent sides of an isosceles triangle are the legs, and the unfit side is called the bottom line.
What are the areas of the isosceles triangles?
The areas of the isosceles triangles are defined as half the product of the bottom line and height of a triangle. The method to calculate the area of an isosceles triangle is (½) bh square unit.
Conclusion.
As previously stated, an isosceles triangle is defined as one with two congruent sides. Additionally, it features two congruent angles. The isosceles triangle theorem’s converse says that a triangle with two equal angles will have two sides.